
It has become, in modern times, a metaphor for the existence of seemingly insignificant moments that alter history and the shape of destiny.
Nearly 45 years ago, during the 139th meeting of the American Association for the Advancement of Science, Edward Lorenz posed a question: “Does the flap of a butterfly’s wings in Brazil set off a tornado in Texas?” The answer to that question probably differs from what you have heard.
The concept referred to as the butterfly effect has been embraced by popular culture, where the term is often used to emphasize the outsize significance of minute occurrences, as in the 1990 movie Havana, in which Robert Redford, playing the role of Jack Weil, a gambler with a knack for math, proclaims to his costar, Lena Olin, that “a butterfly can flutter its wings over a flower in China and cause a hurricane in the Caribbean.”
Lorenz, the mild-mannered Massachusetts Institute of Technology meteorology professor who developed the concept, never intended for it to be applied in this way. Indeed, he meant to convey the opposite point.
The purpose of his provocative question, he said, was to illustrate the idea that some complex dynamical systems exhibit unpredictable behaviors such that small variances in the initial conditions could have profound and widely divergent effects on the system’s outcomes. Because of the sensitivity of these systems, outcomes are unpredictable. This idea became the basis for a branch of mathematics known as chaos theory, which has been applied in countless scenarios since its introduction.
Lorenz’s insight called into question laws introduced as early as 1687 by Sir Isaac Newton suggesting that nature is a probabilistic mechanical system, “a clockwork universe.”
Similarly, Lorenz challenged Pierre-Simon Laplace, who argued that unpredictability has no place in the universe, asserting that if we knew all the physical laws of nature, then “nothing would be uncertain and the future, as the past, would be present to [our] eyes.”
Lorenz discovered that this deterministic interpretation of the universe could not account for the imprecision in human measurement of physical phenomena. He observed that nature’s interdependent cause-and-effect relationships are too complex to resolve. To approximate the most likely outcomes for such complex systems as weather patterns, he began using sets of slightly different starting conditions to conduct parallel meteorological simulations. This method is still used today to generate our daily weather forecasts.
Now, we pose a question: “If we could rerun life from the beginning, would it turn out the same?” In Replaying Evolution, Zachary Blount addresses this question through empirical analyses of the Long-Term Evolution Experiment, an ongoing study in experimental evolution—now approaching 30 years of investigation—that has been tracking genetic changes in several initially identical populations of Escherichia coli bacteria.
He investigates the role of small differences in the evolutionary substrate on the outcome of the process. Interestingly, in Anyone Can Become a Troll Justin Cheng, Cristian Danescu-Niculescu-Mizil, Jure Leskovec, and Michael Bernstein explain that the likelihood ordinary people will cultivate certain online behaviors also depends on a specific set of circumstances, ones that neither guarantee nor exclude the possibility of becoming an aggressor; and in Technologue, Smart and Squishy Robots Yigit Mengüç describes the unique technological conditions under which the development of soft-bodied robots became feasible. Each of these pieces features a complex system—evolutionary, psychological, or technical—that demonstrates the utility of chaos theory for identifying circumstances with unpredictable outcomes.
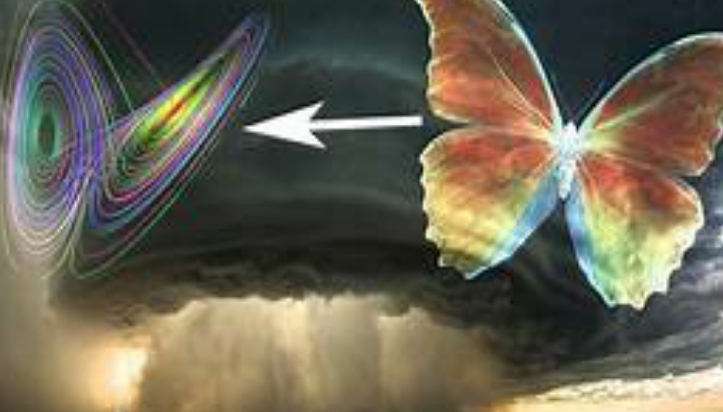
Although Lorenz died in 2008, it is clear that his enduring contribution to our understanding of complex systems deserves celebration—particularly this May 23rd, which would have been his 100th birthday.
Source: JAMIE L. VERNON



















































Comments are closed.